Biophysics Problem 25

What force \(F_m\) would the muscle exert to support the \(10\; kg\) mass? Neglect the mass of the forearm. What force does the bone in the upper arm (humerus) exert on the forearm?

We start off with a diagram showing the essential features of the problem. To this diagram, we will eventually add all forces acting on the forearm.

Draw the diagram now.

diagram of the forces acting on your forearm
Your diagram should look similar to the one above.

Note that this problem involves a number of vertical forces, but no horizontal forces.

 Which forces are involved in this problem?

Gravity pulls down on the mass held in the hand. The muscle pulls up. Also, the vertical bone in the upper arm pushes straight down on the bone of the forearm.

Add these three forces to your diagram.

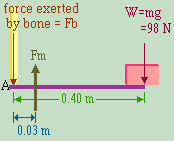
diagram with forces acting on forearm including gravity

Let's label the point where the two bones make contact as '\(A\)'.

Note that all three of the following statements are correct:

  1. The sum of the moments about '\(A\)' is zero.
  2. The sum of the moments about '\(F_m\)' is zero.
  3. The algebraic sum of the three forces is zero.

 However, only one of these statements is useful to us. Which one is it?

Using the first statement will give us an equation with only one unknown in it. (The other two statements would give us two unknowns.)

The force \('{F_b}'\) would not appear in the equation since it passes through the point \('A',\) and therefore exerts no moment or torque about \('A'.\)

Now write out your equation for the moments about  \('A',\) and solve for  \('{F_m}'.\)

Your equation for the moments about \('A'\) should look like this.

\(F_m \times 0.03\;m - 98\;N \times 0.40\; m = 0 \\ F_m = \frac{98\;N \times 0.40\; m}{0.03\;m} \\ = 1307\;N\)

Or approximately, \(F_m = 1310\; N\)

The answer in your text may have been given as  \(F_m = 1330 \;N.\) If so, it was calculated by a lazy author, who used  \(g = 10 \;m/s.\)

 Now that you know  \('{F_m}'\)  you should be able to calculate \('{F_b}'\)  with no difficulty. Do the calculation now.

There are only three vertical forces, as we discussed earlier. You know two of them, and also that they must add up algebraically to zero.

Your equation should look like this:

\(F_b + W + F_m = 0 \\ F_b - 98\; N + 1307\; N = 0 \\ F_b = 1209\; N\)

Or approximately, \(F_b = 1210\; N.\)